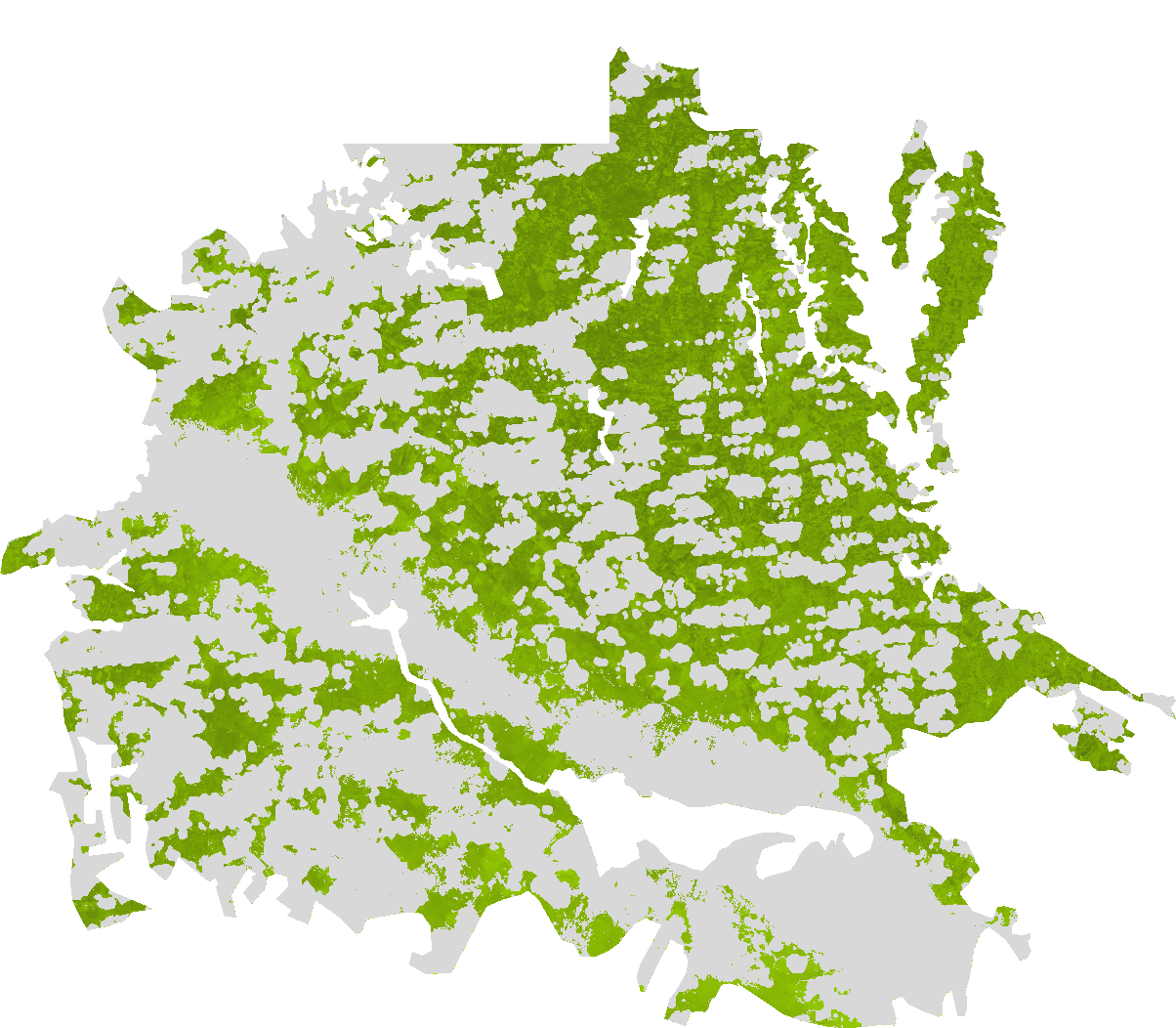
The Green Normalized Difference Vegetation Index algorithm was developed by Gitelson et al. (1996). The authors verified that GNDVI was more sensible than NDVI to identify different concentration rates of chlorophyll which is highly correlated at nitrogen. The use of green spectral band was more efficient than the red spectral band to discriminate nitrogen. The GNDVI results from the following equation: GNDVI = (IR_factor * near_IR - green_factor * green) / (IR_factor * near_IR + green_factor * green)

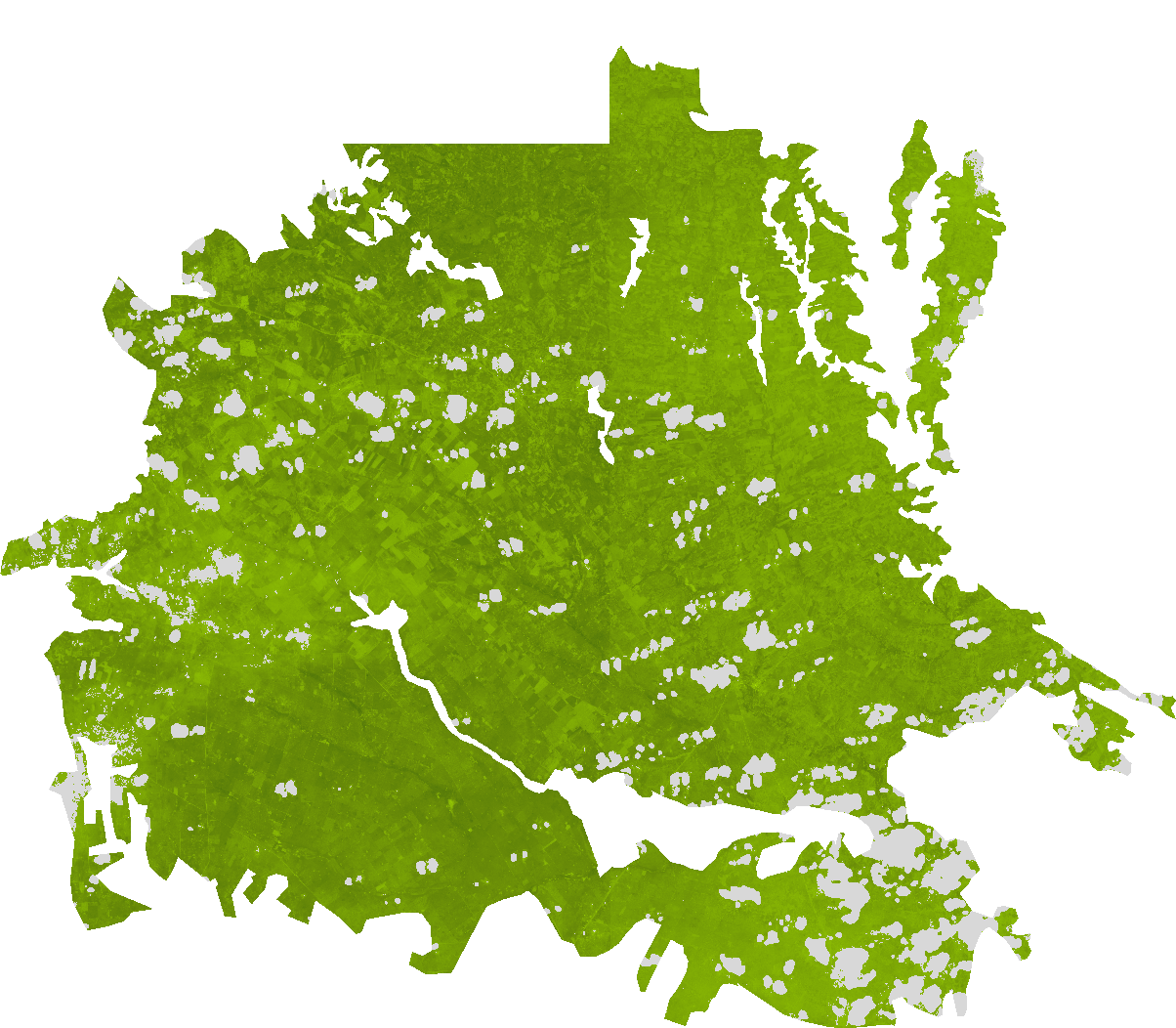
The Green Normalized Difference Vegetation Index algorithm was developed by Gitelson et al. (1996). The authors verified that GNDVI was more sensible than NDVI to identify different concentration rates of chlorophyll which is highly correlated at nitrogen. The use of green spectral band was more efficient than the red spectral band to discriminate nitrogen. The GNDVI results from the following equation: GNDVI = (IR_factor * near_IR - green_factor * green) / (IR_factor * near_IR + green_factor * green)

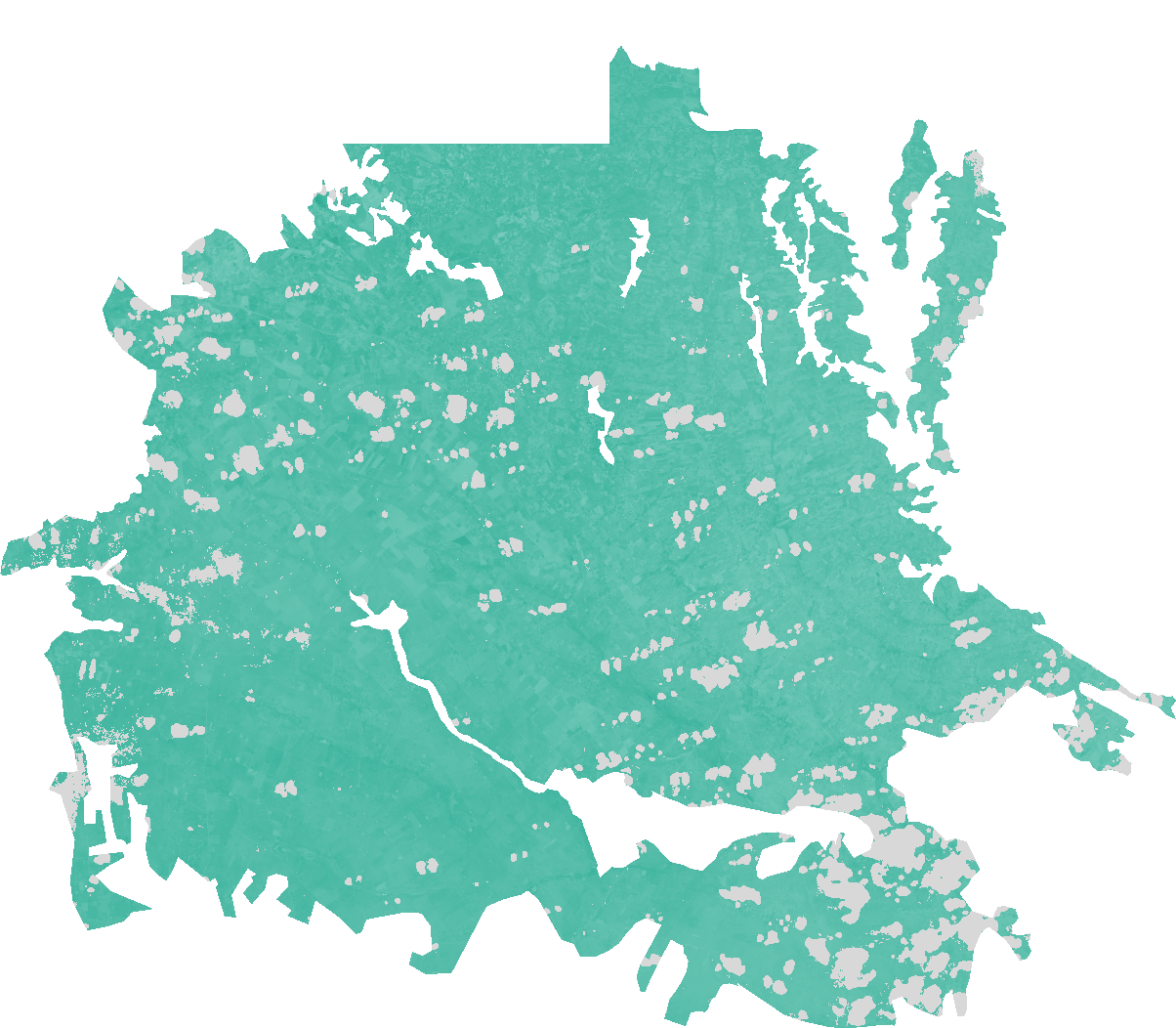
The Normalized Difference Water Index algorithm was developed by Gao (19964) being a measure of liquid water molecules in vegetation canopies that interacted with the incoming solar radiation. NDWI is sensitive to changes in liquid water content of vegetation canopies. It is less sensitive to atmospheric effects than NDVI. NDWI does not remove completely the background soil reflectance effects therefore it should be considered as an independent vegetation index. It is complementary to not a substitute for NDVI. The NDWI results from the following equation: NDWI = (IR_factor * near_IR - mir_factor * middle_IR) / (IR_factor * near_IR + mir_factor * middle_IR)

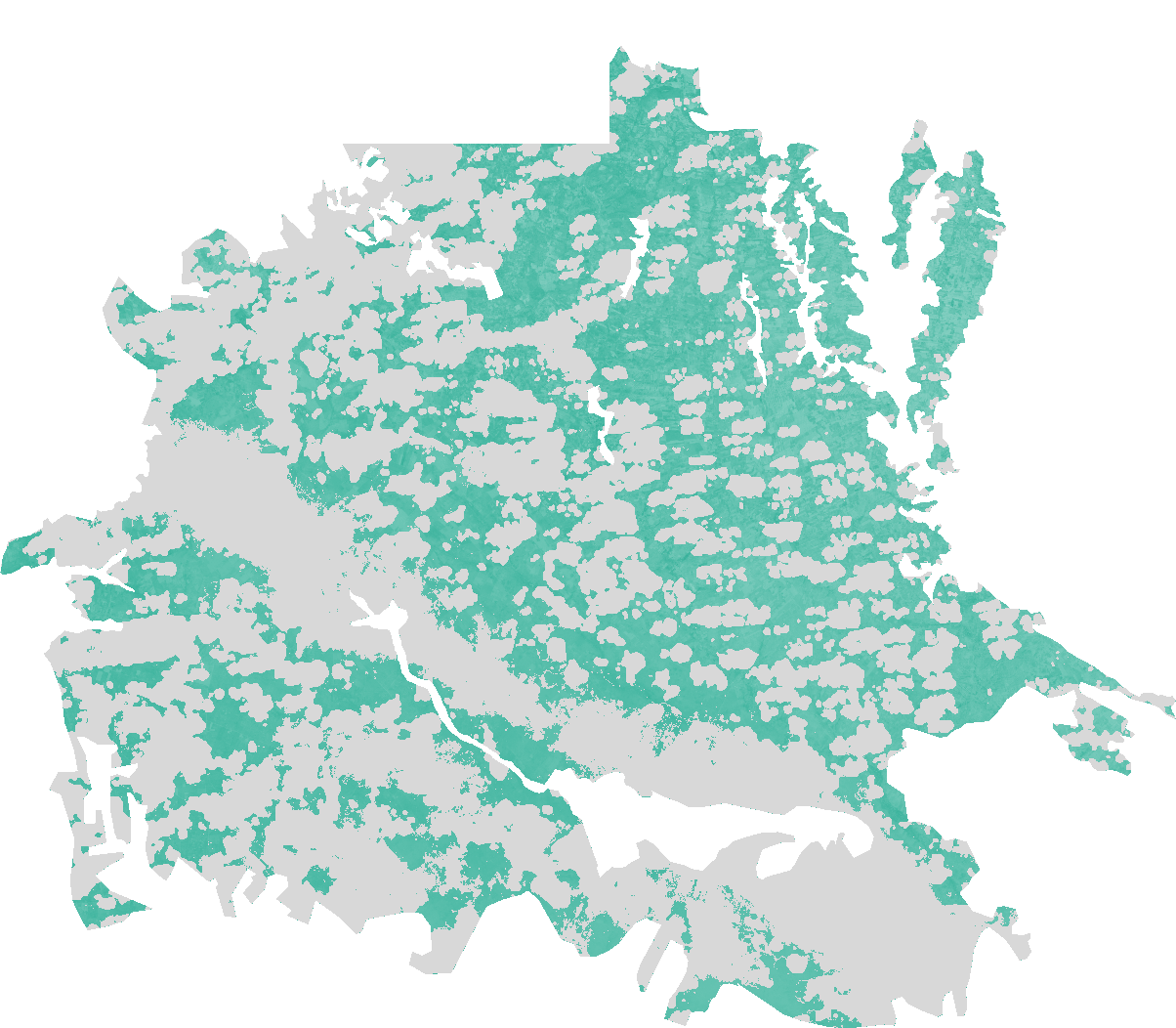
The Normalized Difference Water Index algorithm was developed by Gao (19964) being a measure of liquid water molecules in vegetation canopies that interacted with the incoming solar radiation. NDWI is sensitive to changes in liquid water content of vegetation canopies. It is less sensitive to atmospheric effects than NDVI. NDWI does not remove completely the background soil reflectance effects therefore it should be considered as an independent vegetation index. It is complementary to not a substitute for NDVI. The NDWI results from the following equation: NDWI = (IR_factor * near_IR - mir_factor * middle_IR) / (IR_factor * near_IR + mir_factor * middle_IR)

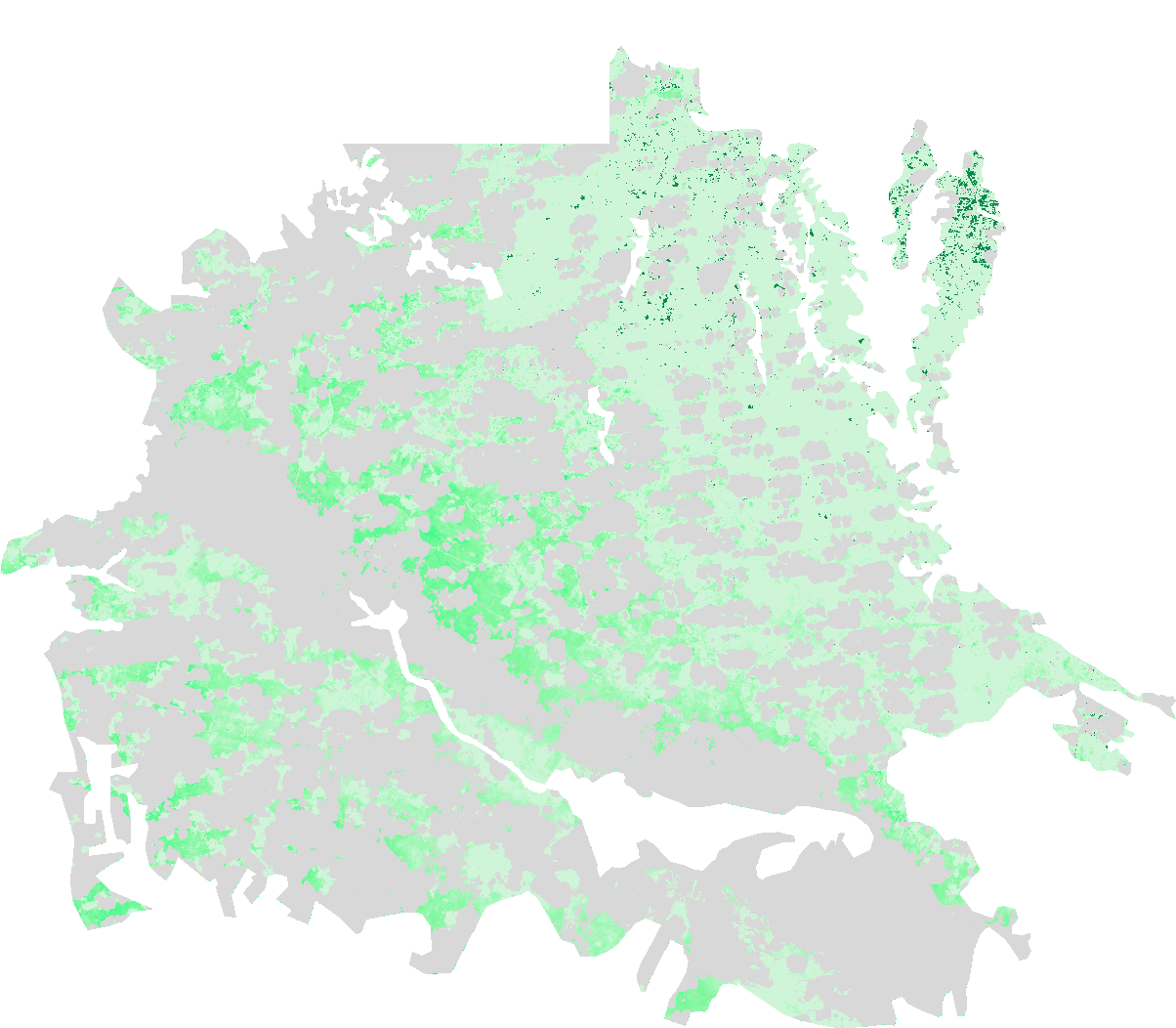
The Transformed Soil Adjusted Vegetation Index (TSAVI) method is a vegetation index that minimizes soil brightness influences by assuming the soil line has an arbitrary slope and intercept. TSAVI = (s *(NIR - s * Red - a)) / (a * NIR + Red - a * s + X * (1 + s2))

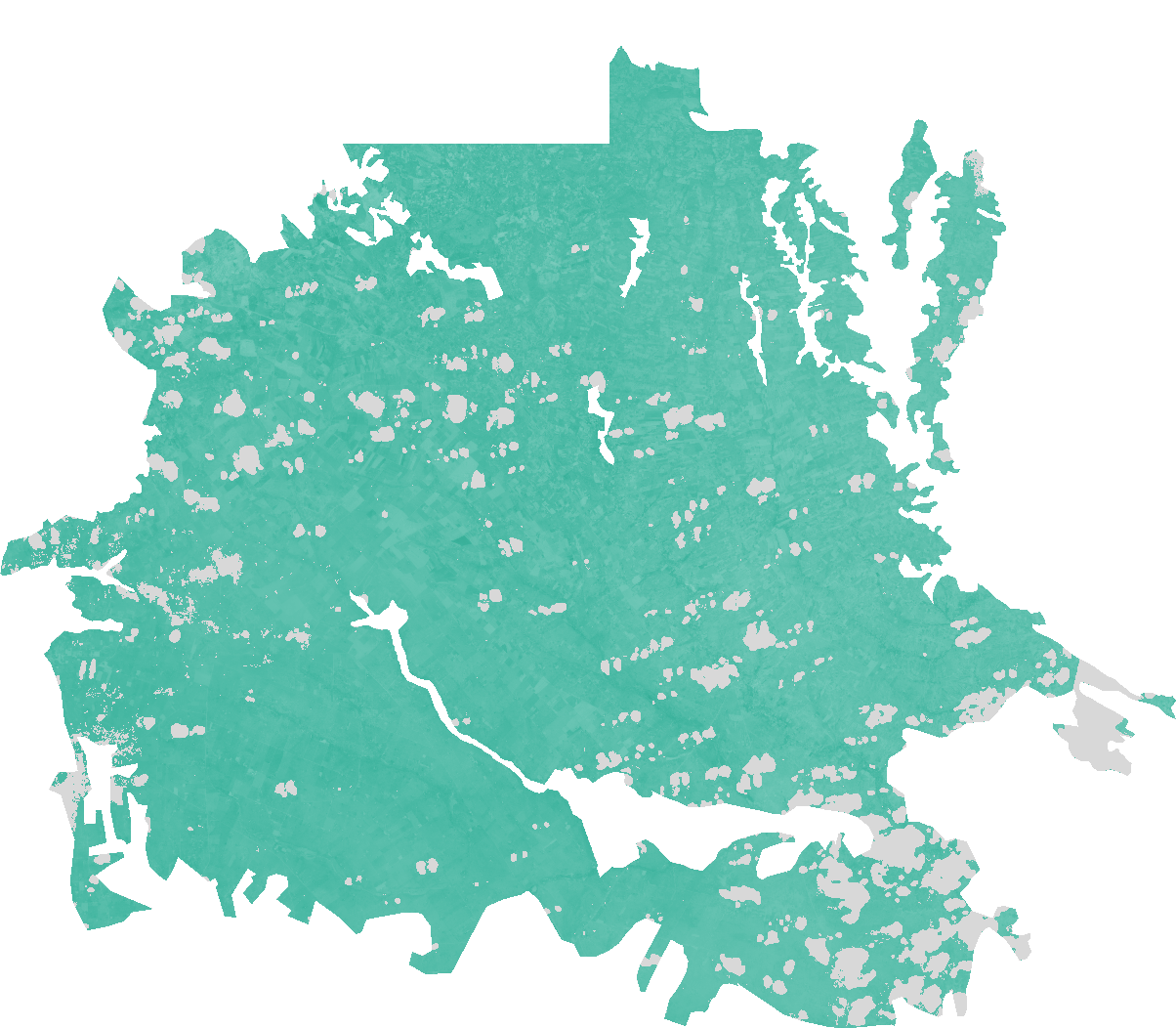
The Normalized Difference Water Index algorithm was developed by Gao (19964) being a measure of liquid water molecules in vegetation canopies that interacted with the incoming solar radiation. NDWI is sensitive to changes in liquid water content of vegetation canopies. It is less sensitive to atmospheric effects than NDVI. NDWI does not remove completely the background soil reflectance effects therefore it should be considered as an independent vegetation index. It is complementary to not a substitute for NDVI. The NDWI results from the following equation: NDWI = (IR_factor * near_IR - mir_factor * middle_IR) / (IR_factor * near_IR + mir_factor * middle_IR)

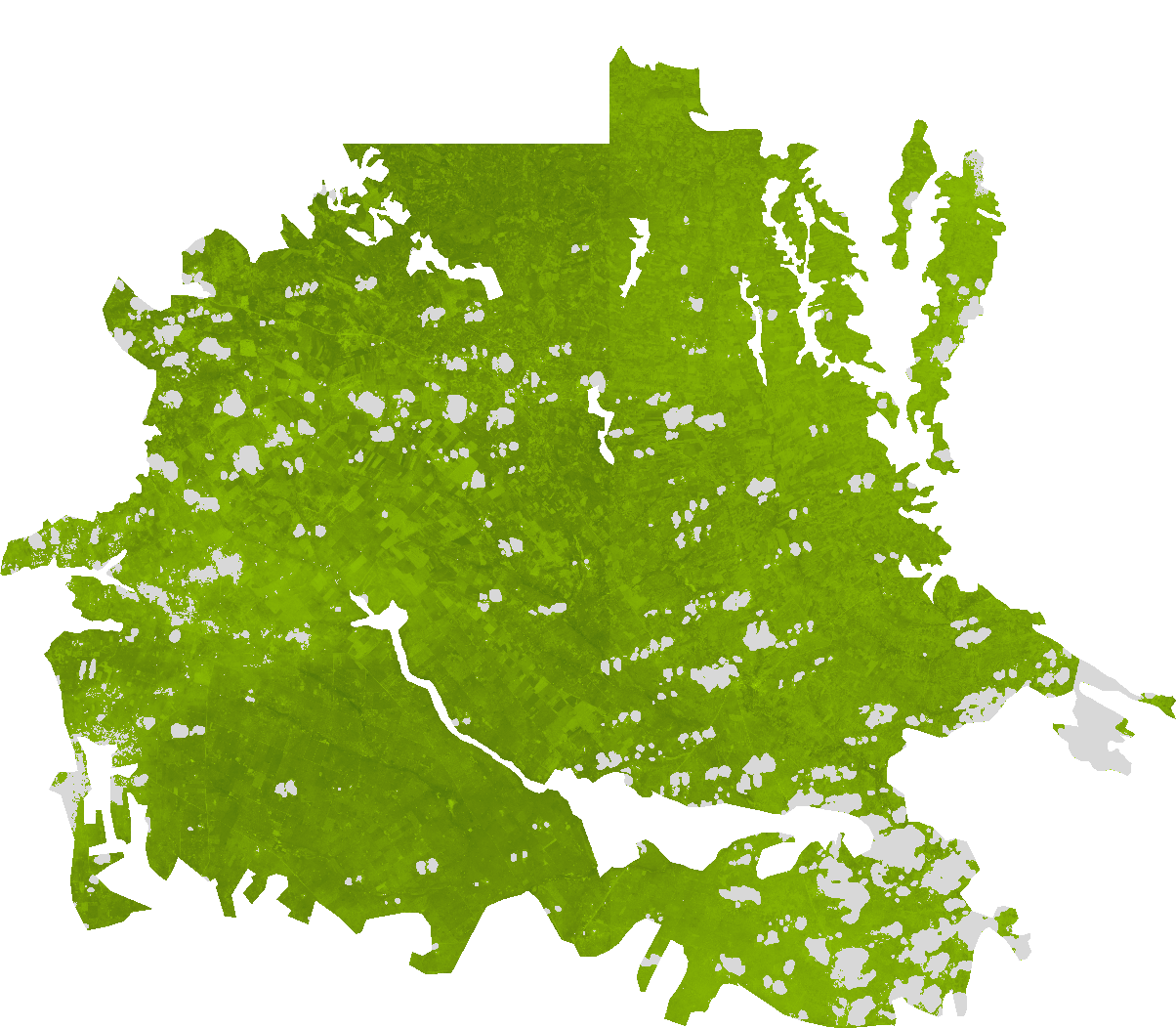
The Green Normalized Difference Vegetation Index algorithm was developed by Gitelson et al. (1996). The authors verified that GNDVI was more sensible than NDVI to identify different concentration rates of chlorophyll which is highly correlated at nitrogen. The use of green spectral band was more efficient than the red spectral band to discriminate nitrogen. The GNDVI results from the following equation: GNDVI = (IR_factor * near_IR - green_factor * green) / (IR_factor * near_IR + green_factor * green)

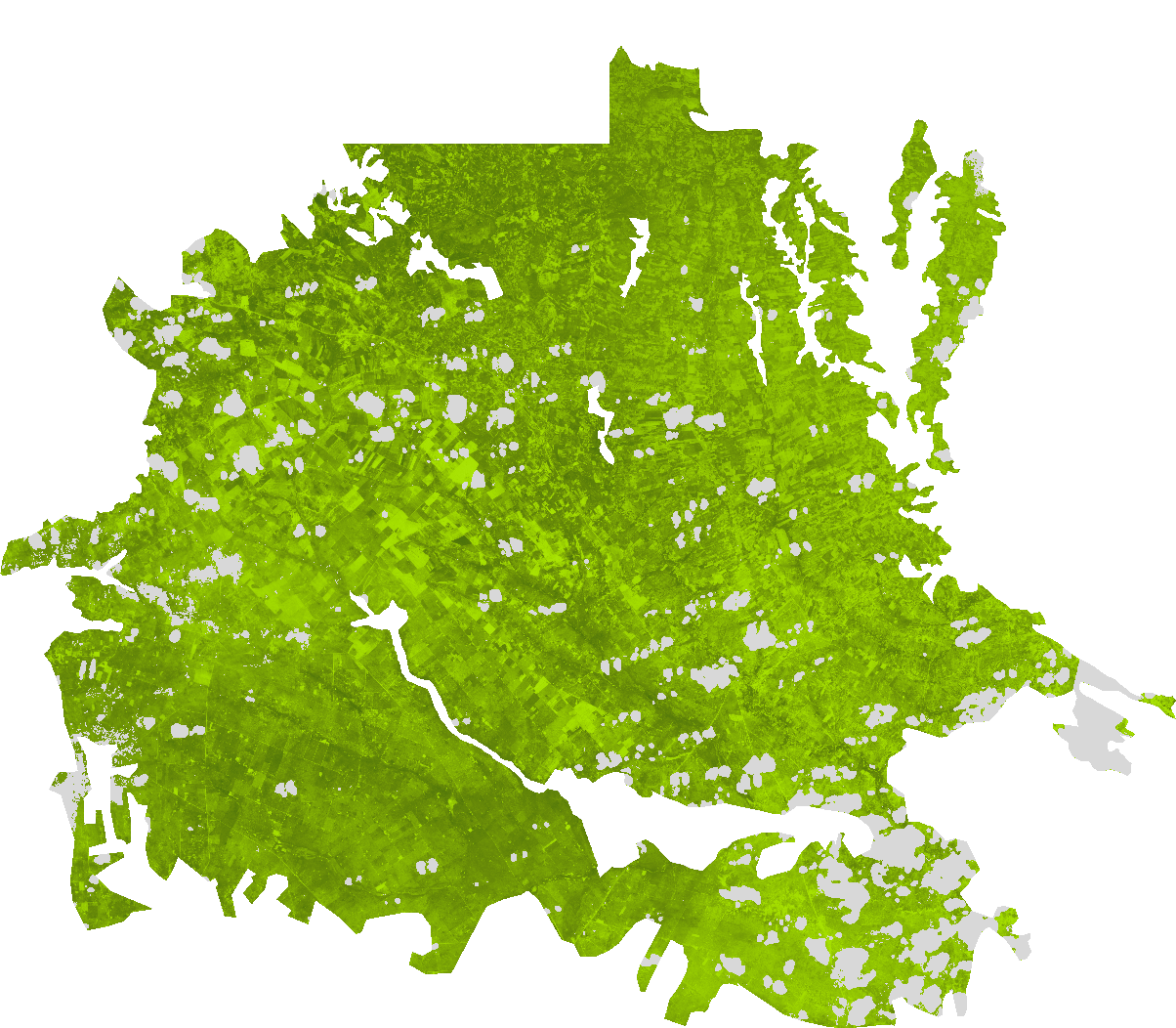
Atmospherically Resistant Vegetation Index: This index takes advantage of the different scattering responses from the blue and red band to retrieve information regarding the atmosphere opacity. The Atmospherically Resistant Vegetation Index algorithm was introduced by Kaufman and Tanre (1992). The resistance of the ARVI to atmospheric effects (in comparison to the NDVI) is accomplished by a self-correction process for the atmospheric effect on the red channel. This is done using the difference in the radiance between the blue and the red channels to correct the radiance in the red channel. Compared to the red band the blue band is much more easily scattered by the atmosphere particles. This explains why the sky is usually perceived as being blue. Thus the ARVI index takes advantage of the different scattering responses from the blue and red band to retrieve information regarding the atmosphere opacity. Simulations using radiative transfer computations on arithmetic and natural surface spectra for various atmospheric conditions show that ARVI has a similar dynamic range to the NDVI but is on average four times less sensitive to atmospheric effects than the NDVI. The ARVI results from the following equation:ARVI = (IR_factor * near_IR - rb) / (IR_factor * near_IR + rb) where: rb = (red_factor * red) - gamma * (blue_factor * blue - red_factor * red) with gamma = 1. The main reason why the blue band is more susceptible to atmospheric scattering than the red band is because its wavelength is shorter. Generally the shorter wavelength has stronger scattering. It's very similar to the way sea waves behave over oceans. When a large wave strikes an object such as a ferryboat it is more capable of continuing on its path by going around the object. On the other hand it is dispersed more easily when the waves are smaller in size. Consequently by obtaining the difference between the reflectance of the highly sensitive blue band and the less sensitive red band (blue - red) it serves like an indicator of what the atmospheric conditions were like. Here gamma serves as a weighting function for the difference reflectance of the two bands. Various values can be chosen for it which mainly depends on the type of aerosol size. According to Kaufaman and Tanre's statement in 1992 it is best to select a gamma value of 1 when information on the aerosol type is not available. Consequently the main purpose of the above rb equation is to decrease the influence brought forth from the atmosphere where a more accurate assessment of the value of the red reflectance can be obtained.

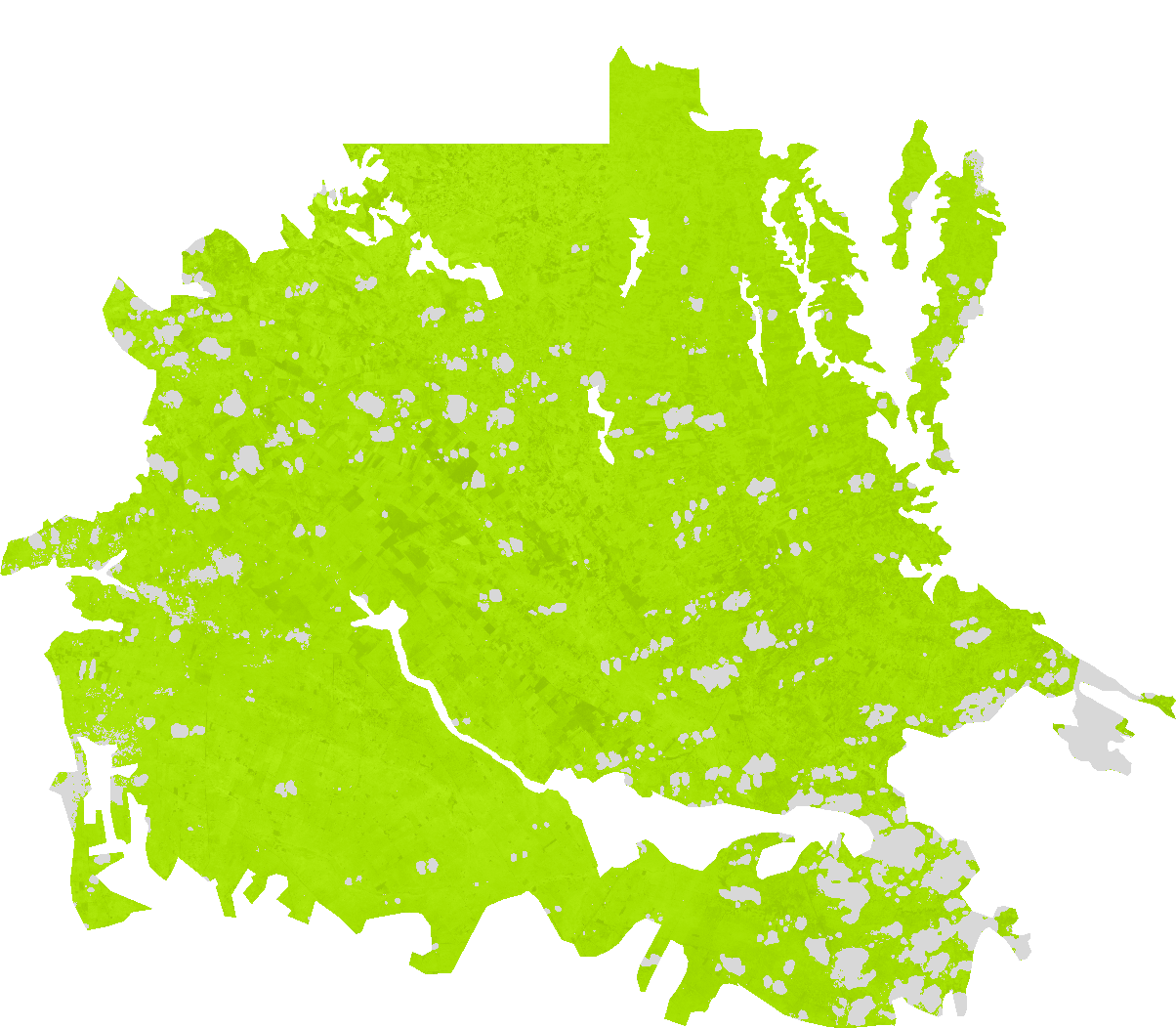
The Normalised Difference Turbidity Index (NDTI) quantifies the difference in reflectance between specific spectral bands which correlates with suspended sediment and turbidity levels.

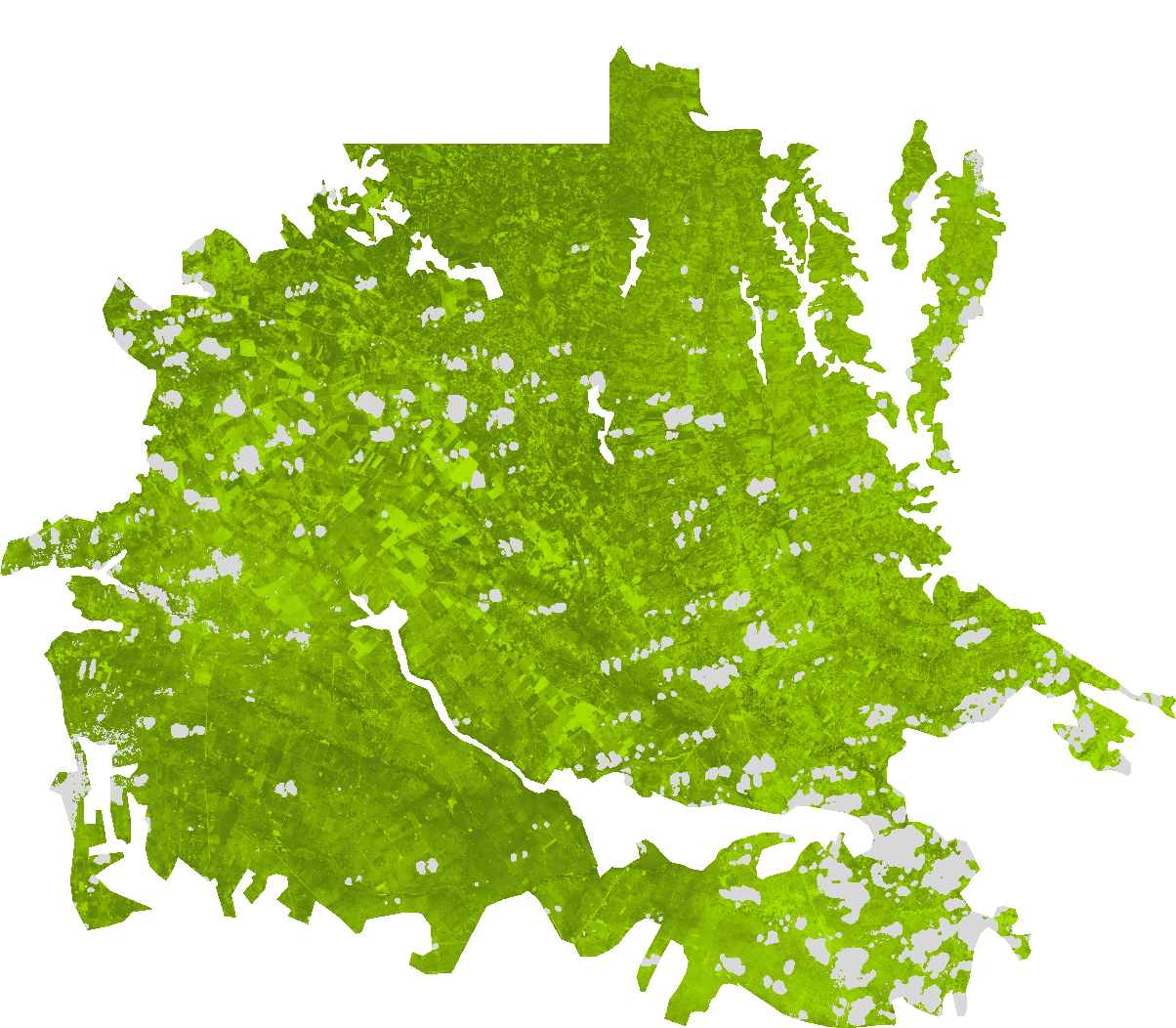
Atmospherically Resistant Vegetation Index: This index takes advantage of the different scattering responses from the blue and red band to retrieve information regarding the atmosphere opacity. The Atmospherically Resistant Vegetation Index algorithm was introduced by Kaufman and Tanre (1992). The resistance of the ARVI to atmospheric effects (in comparison to the NDVI) is accomplished by a self-correction process for the atmospheric effect on the red channel. This is done using the difference in the radiance between the blue and the red channels to correct the radiance in the red channel. Compared to the red band the blue band is much more easily scattered by the atmosphere particles. This explains why the sky is usually perceived as being blue. Thus the ARVI index takes advantage of the different scattering responses from the blue and red band to retrieve information regarding the atmosphere opacity. Simulations using radiative transfer computations on arithmetic and natural surface spectra for various atmospheric conditions show that ARVI has a similar dynamic range to the NDVI but is on average four times less sensitive to atmospheric effects than the NDVI. The ARVI results from the following equation:ARVI = (IR_factor * near_IR - rb) / (IR_factor * near_IR + rb) where: rb = (red_factor * red) - gamma * (blue_factor * blue - red_factor * red) with gamma = 1. The main reason why the blue band is more susceptible to atmospheric scattering than the red band is because its wavelength is shorter. Generally the shorter wavelength has stronger scattering. It's very similar to the way sea waves behave over oceans. When a large wave strikes an object such as a ferryboat it is more capable of continuing on its path by going around the object. On the other hand it is dispersed more easily when the waves are smaller in size. Consequently by obtaining the difference between the reflectance of the highly sensitive blue band and the less sensitive red band (blue - red) it serves like an indicator of what the atmospheric conditions were like. Here gamma serves as a weighting function for the difference reflectance of the two bands. Various values can be chosen for it which mainly depends on the type of aerosol size. According to Kaufaman and Tanre's statement in 1992 it is best to select a gamma value of 1 when information on the aerosol type is not available. Consequently the main purpose of the above rb equation is to decrease the influence brought forth from the atmosphere where a more accurate assessment of the value of the red reflectance can be obtained.