The Pigment Specific Simple Ratio (chlorophyll index) algorithm was developed by Blackburn (1998). It investigates the potential of a range of spectral approaches for quantifying pigments at the scale of the whole plant canopy. When applying existing narrow-band pigment indices the PSSR algorithms have the strongest and most linear relationships with canopy concentration per unit area of Chl a (Chlorophyll a) Chl b (Chlorophyll b) and Cars (carotenoids). The PSSRa results from the following equation: PSSRa = (IR_factor * near_IR) / (red_factor * red)

Colour Index: The Colour Index algorithm was developed to differentiate soils in the field. Low valued CIs have been shown to be correlated with the presence of a high concentration of carbonates or sulfates and higher values to be correlated with crusted soils and sands in arid regions (Escadfal 1989). In most cases the CI gives complementary information with the BI and the NDVI. Used for diachronic analyses they help for a better understanding of the evolution of soil surfaces. The CI results from the following equation: CI = (red_factor * red - green_factor * green) / (red_factor * red + green_factor * green)

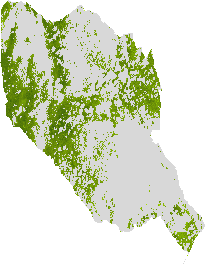
The fraction of absorbed photosynthetically active radiation (FAPAR sometimes also noted fAPAR or fPAR) is the fraction of the incoming solar radiation in the photosynthetically active radiation spectral region that is absorbed by a photosynthetic organism typically describing the light absorption across an integrated plant canopy. This biophysical variable is directly related to the primary productivity of photosynthesis and some models use it to estimate the assimilation of carbon dioxide in vegetation in conjunction with the leaf area index. FAPAR can also be used as an indicator of the state and evolution of the vegetation cover with this function it advantageously replaces the Normalized Difference Vegetation Index (NDVI) provided it is itself properly estimated.

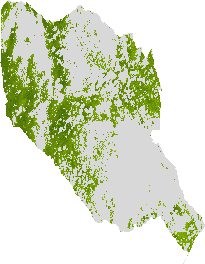
The fraction of absorbed photosynthetically active radiation (FAPAR sometimes also noted fAPAR or fPAR) is the fraction of the incoming solar radiation in the photosynthetically active radiation spectral region that is absorbed by a photosynthetic organism typically describing the light absorption across an integrated plant canopy. This biophysical variable is directly related to the primary productivity of photosynthesis and some models use it to estimate the assimilation of carbon dioxide in vegetation in conjunction with the leaf area index. FAPAR can also be used as an indicator of the state and evolution of the vegetation cover with this function it advantageously replaces the Normalized Difference Vegetation Index (NDVI) provided it is itself properly estimated.

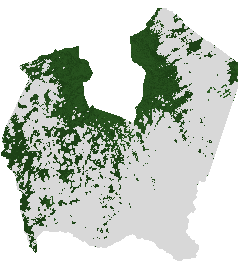
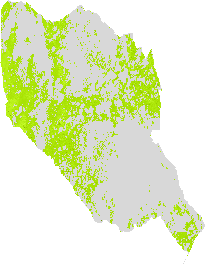
The Transformed Normalized Difference Vegetation Index algorithm indicates a relation between the amount of green biomass that is found in a pixel. (Senseman et.al. 1996). Transformed Normalised Difference Vegetation index (TNDVI) is the square root of the NDVI. It has higher coefficient of determination for the same variable and this is the difference between TNDVI and NDVI. The formula of TNDVI has always positive values and the variances of the ratio are proportional to mean values. The TNDVI results from the following equation: TNDVI = sqrt( (IR_factor * near_IR - red_factor * red) / (IR_factor * near_IR + red_factor * red) + 0.5)

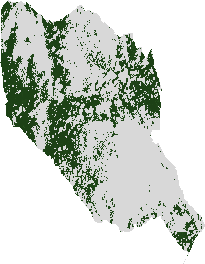
The Transformed Normalized Difference Vegetation Index algorithm indicates a relation between the amount of green biomass that is found in a pixel. (Senseman et.al. 1996). Transformed Normalised Difference Vegetation index (TNDVI) is the square root of the NDVI. It has higher coefficient of determination for the same variable and this is the difference between TNDVI and NDVI. The formula of TNDVI has always positive values and the variances of the ratio are proportional to mean values. The TNDVI results from the following equation: TNDVI = sqrt( (IR_factor * near_IR - red_factor * red) / (IR_factor * near_IR + red_factor * red) + 0.5)

The Transformed Normalized Difference Vegetation Index algorithm indicates a relation between the amount of green biomass that is found in a pixel. (Senseman et.al. 1996). Transformed Normalised Difference Vegetation index (TNDVI) is the square root of the NDVI. It has higher coefficient of determination for the same variable and this is the difference between TNDVI and NDVI. The formula of TNDVI has always positive values and the variances of the ratio are proportional to mean values. The TNDVI results from the following equation: TNDVI = sqrt( (IR_factor * near_IR - red_factor * red) / (IR_factor * near_IR + red_factor * red) + 0.5)

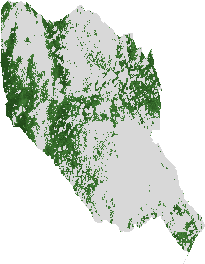
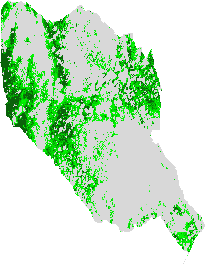
The Normalized Difference Vegetation Index (NDVI) is a measure of the amount and vigor of vegetation on the land surface and NDVI spatial composite images are developed to more easily distinguish green vegetation from bare soils. In general NDVI values range from -1.0 to 1.0 with negative values indicating clouds and water positive values near zero indicating bare soil and higher positive values of NDVI ranging from sparse vegetation (0.1 - 0.5) to dense green vegetation (0.6 and above).

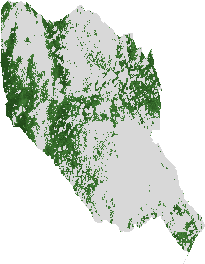
The Normalized Difference Vegetation Index (NDVI) is a measure of the amount and vigor of vegetation on the land surface and NDVI spatial composite images are developed to more easily distinguish green vegetation from bare soils. In general NDVI values range from -1.0 to 1.0 with negative values indicating clouds and water positive values near zero indicating bare soil and higher positive values of NDVI ranging from sparse vegetation (0.1 - 0.5) to dense green vegetation (0.6 and above).

The Red-Edge Inflection Point Index algorithm was developed for applications in biomass and nitrogen (N) uptake measurement/management in heterogeneous fields.- Guyot et al. (1988). Red edge as the inflection point of the strong red absorption to near infrared reflectance includes the information of both crop N and growth status. The reflectance around red edge is sensitive to wide range of crop chlorophyll content N content LAI and biomass (Hatfield et al. 2008 Mutanga and Skidmore 2007 Steele et al. 2008b). The REIP general formula is based on linear four-point interpolation technique and it uses four wavebands (670 700 740 and 780 nm) - Guyot and Baret (1988). The REIP results from the following (Sensor-dependent) equation: REIP = 700 + 40 * ((r670 + r780)/2 - r700) / (r740 - r700) - as general formula or: REIP = 700 + 40 * ( (red1_factor * red1 + IR_factor * near_IR)/2) - red2_factor * red2 ) / (red3_factor * red3 - red2_factor * red2) )