The Pigment Specific Simple Ratio (chlorophyll index) algorithm was developed by Blackburn (1998). It investigates the potential of a range of spectral approaches for quantifying pigments at the scale of the whole plant canopy. When applying existing narrow-band pigment indices the PSSR algorithms have the strongest and most linear relationships with canopy concentration per unit area of Chl a (Chlorophyll a) Chl b (Chlorophyll b) and Cars (carotenoids). The PSSRa results from the following equation: PSSRa = (IR_factor * near_IR) / (red_factor * red)

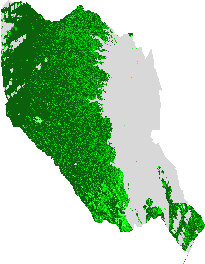
The Pigment Specific Simple Ratio (chlorophyll index) algorithm was developed by Blackburn (1998). It investigates the potential of a range of spectral approaches for quantifying pigments at the scale of the whole plant canopy. When applying existing narrow-band pigment indices the PSSR algorithms have the strongest and most linear relationships with canopy concentration per unit area of Chl a (Chlorophyll a) Chl b (Chlorophyll b) and Cars (carotenoids). The PSSRa results from the following equation: PSSRa = (IR_factor * near_IR) / (red_factor * red)

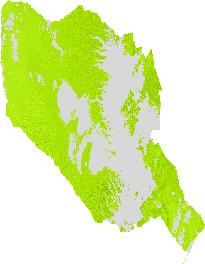
The Normalised Difference Turbidity Index (NDTI) quantifies the difference in reflectance between specific spectral bands which correlates with suspended sediment and turbidity levels.

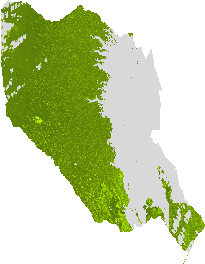
The Normalized Burn Ratio Index (NBR) uses the NIR and SWIR bands to emphasize burned areas while mitigating illumination and atmospheric effects. NBR = (NIR - SWIR) / (NIR+ SWIR)

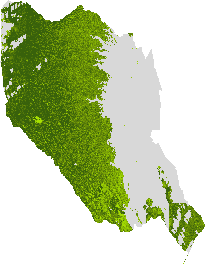
Inverted Red-Edge Chlorophyll Index: The Inverted Red-Edge Chlorophyll Index algorithm incorporates the reflectance in four bands to estimate canopy chlorophyll content (Guyot and Baret 1988 Clevers et al. 2000). The 'red edge' is the name given to the abrupt reflectance change in the 680-740 nm region of vegetation spectra that is caused by the combined effects of strong chlorophyll absorption and leaf internal scattering. Increases in the amount of chlorophyll visible to the sensor either through an increase in leaf chlorophyll content or Leaf Area Index (LAI) result in a broadening of a major chlorophyll absorption feature centred around 680 nm. The effect is to cause a movement of the point of maximum slope termed the red edge position (REP). The position of the red edge has been used as an indicator of stress and senescence of vegetation (Collins1978 Horler et al. 1983 Rock et al. 1988 Boochs et al. 1990 Jago and Curran 1995). The IRECI results from the following (Sensor-dependent) equation: IRECI = (IR_factor * near_IR - red1_factor * red1) / (red2_factor * red2 / red3_factor * red3). For Sentinel-2 the formula is: (B7 - B4) / (B5 / B6) where (Central wavelength/Bandwidth): B7 = 783 nm (15 nm) B6 = 740 nm (15 nm) B5 = 705 nm (15 nm) B4 = 665 nm (30 nm)

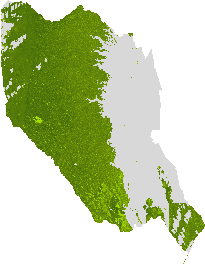
The fraction of absorbed photosynthetically active radiation (FAPAR sometimes also noted fAPAR or fPAR) is the fraction of the incoming solar radiation in the photosynthetically active radiation spectral region that is absorbed by a photosynthetic organism typically describing the light absorption across an integrated plant canopy. This biophysical variable is directly related to the primary productivity of photosynthesis and some models use it to estimate the assimilation of carbon dioxide in vegetation in conjunction with the leaf area index. FAPAR can also be used as an indicator of the state and evolution of the vegetation cover with this function it advantageously replaces the Normalized Difference Vegetation Index (NDVI) provided it is itself properly estimated.

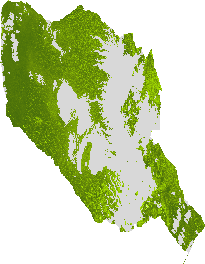
Atmospherically Resistant Vegetation Index: This index takes advantage of the different scattering responses from the blue and red band to retrieve information regarding the atmosphere opacity. The Atmospherically Resistant Vegetation Index algorithm was introduced by Kaufman and Tanre (1992). The resistance of the ARVI to atmospheric effects (in comparison to the NDVI) is accomplished by a self-correction process for the atmospheric effect on the red channel. This is done using the difference in the radiance between the blue and the red channels to correct the radiance in the red channel. Compared to the red band the blue band is much more easily scattered by the atmosphere particles. This explains why the sky is usually perceived as being blue. Thus the ARVI index takes advantage of the different scattering responses from the blue and red band to retrieve information regarding the atmosphere opacity. Simulations using radiative transfer computations on arithmetic and natural surface spectra for various atmospheric conditions show that ARVI has a similar dynamic range to the NDVI but is on average four times less sensitive to atmospheric effects than the NDVI. The ARVI results from the following equation:ARVI = (IR_factor * near_IR - rb) / (IR_factor * near_IR + rb) where: rb = (red_factor * red) - gamma * (blue_factor * blue - red_factor * red) with gamma = 1. The main reason why the blue band is more susceptible to atmospheric scattering than the red band is because its wavelength is shorter. Generally the shorter wavelength has stronger scattering. It's very similar to the way sea waves behave over oceans. When a large wave strikes an object such as a ferryboat it is more capable of continuing on its path by going around the object. On the other hand it is dispersed more easily when the waves are smaller in size. Consequently by obtaining the difference between the reflectance of the highly sensitive blue band and the less sensitive red band (blue - red) it serves like an indicator of what the atmospheric conditions were like. Here gamma serves as a weighting function for the difference reflectance of the two bands. Various values can be chosen for it which mainly depends on the type of aerosol size. According to Kaufaman and Tanre's statement in 1992 it is best to select a gamma value of 1 when information on the aerosol type is not available. Consequently the main purpose of the above rb equation is to decrease the influence brought forth from the atmosphere where a more accurate assessment of the value of the red reflectance can be obtained.

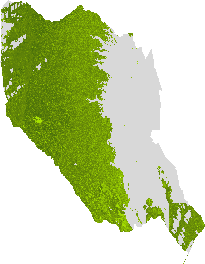
Enhanced vegetation index: In areas of dense canopy cover where leaf area index (LAI) is high the blue wavelengths can be used to improve the accuracy of NDVI as it corrects for soil background signals and atmospheric influences. Values description: The range of values for EVI is -1 to 1 with healthy vegetation generally around 0.20 to 0.80.

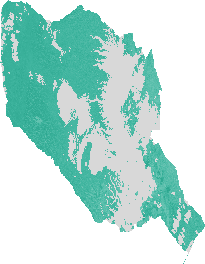
The Normalized Difference Water Index algorithm was developed by Gao (19964) being a measure of liquid water molecules in vegetation canopies that interacted with the incoming solar radiation. NDWI is sensitive to changes in liquid water content of vegetation canopies. It is less sensitive to atmospheric effects than NDVI. NDWI does not remove completely the background soil reflectance effects therefore it should be considered as an independent vegetation index. It is complementary to not a substitute for NDVI. The NDWI results from the following equation: NDWI = (IR_factor * near_IR - mir_factor * middle_IR) / (IR_factor * near_IR + mir_factor * middle_IR)

The fraction of absorbed photosynthetically active radiation (FAPAR sometimes also noted fAPAR or fPAR) is the fraction of the incoming solar radiation in the photosynthetically active radiation spectral region that is absorbed by a photosynthetic organism typically describing the light absorption across an integrated plant canopy. This biophysical variable is directly related to the primary productivity of photosynthesis and some models use it to estimate the assimilation of carbon dioxide in vegetation in conjunction with the leaf area index. FAPAR can also be used as an indicator of the state and evolution of the vegetation cover with this function it advantageously replaces the Normalized Difference Vegetation Index (NDVI) provided it is itself properly estimated.